Introduction

Integration is area under the curve.

Consider the following diagram:

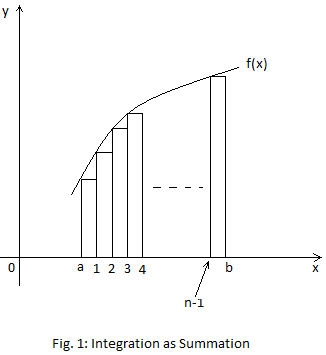
                y = f(x)

You might have seen the following:

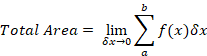
This statement is not perfect. It should actually be:

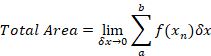
where f(x_n) is a particular height from the curve to the x-axis.

My Discovery

The widths of the vertical strips are equal.

As 𝛿x turns to 0, the number of strips increases.

n is the total number of strips.

As 𝛿x → 0, n → ∞.

The series and the formula for its summation, for all the heights of the strips from a to b, is obtained. If you do not know the formula for the series, develop one, and that may take time. Summing can be done for the heights, from 0 to n-1.

The summation expression above in the statement, is replaced with the summation formula.

As n → ∞, the right-hand-side of the statement becomes the definite integral; and that concludes the scheme.

Let total area be represented by A. So, my statement for the Integration from First Principles is:

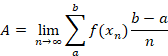
Integration of the Constant, C

Let the definite integral be represented by A, and let the indefinite integral be represented by I. Let the summation expression (discrete summation) be S_n.

Here,

    y = C

i.e.

    f(x) = C

S_n = (C₀ + C + C + C + - - - + C_n-1)𝛿x

     = (nC) 𝛿x

But

⸫ S_n =

The n_s cancel:

=> S_n = C(b - a)

=> Cb - Ca

As n → ∞,

A = Cb -Ca ………………….the definite integral

If a is 0 and b is x,

I = Cx ………………………..the indefinite integral

Integration of y = x

That is,

    f(x) = x

S_n = [a + (a+𝛿x) + (a+2𝛿x) + (a+3𝛿x) + - - - + (a+(n-1)𝛿x)]𝛿x

The series in square brackets is an Arithmetic Progression, with first term = a and common difference = 𝛿x.

But

As n → ∞,

If a is 0 and b is x,

Integration of y=x² 

That is,

        f(x) = x²

S_n = [(a+0𝛿x)² + (a+1𝛿x)² + (a+2𝛿x)² + (a+3𝛿x)² + - - - + (a+(n-1)𝛿x)²]𝛿x

=> S_n = [a²

                 + a² + 2a𝛿x + 𝛿²x

                 + a² + 4a𝛿x + 4𝛿²x

                 + a² + 6a𝛿x + 9𝛿²x

                 +

                  .

                  .

                 + a² + 2a(n-1)𝛿x + (n-1)²𝛿²x]𝛿x

=> S_n = [a² + a² + a² + a² + - - - +a^a_n

                   + 2a𝛿x + 4a𝛿x + 6a𝛿x + - - - + 2a(n-1)𝛿x

                   + 1𝛿²x + 4𝛿²x + 9𝛿²x + - - - + (n-1)²𝛿²x]𝛿x

The first row in square brackets gives, na².

The second row is an Arithmetic Progression, with first term = 0 and common difference = 2a𝛿x, giving,

The third row is,

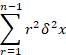
A common formula for the r² series is,

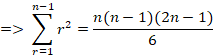
But

As n → ∞,

After subtractions, we have,

If a is 0 and b is x,

Integration of y=x³ 

That is,

        f(x) = x³

S_n = [(a+0𝛿x)³ + (a+1𝛿x)³ + (a+2𝛿x)³ + (a+3𝛿x)³ + - - - + (a+(n-1)𝛿x)³]𝛿x

=> S_n = [a³

                 + a³ + 3a²𝛿x + 3a𝛿²x + 𝛿³x

                 + a³ + 3(2¹)a²𝛿x + 3(2²)a𝛿²x + (2³)𝛿³x

                 + a³ + 3(3¹)a²𝛿x + 3(3²)a𝛿²x + (3³)𝛿³x

                 +

                  .

                  .

                 + a³ + 3(n-1)a²𝛿x + 3(n-1)²a𝛿²x + (n-1)³𝛿³x]𝛿x

=> S_n = [a³ + a³ + a³ + a³ + - - - +a³_n

                   + 3a²𝛿x + 3(3¹)a²𝛿x + 3(3¹)a²𝛿x + - - - + 3(n-1)a²𝛿x

                   + 3a𝛿²x + 3(2²)a𝛿²x + 3(3²)a𝛿²x + - - - + 3(n-1)²a𝛿²x

                   + 𝛿³x + (2³)𝛿³x + (3³)𝛿³x + - - - + (n-1)³𝛿³x]𝛿x

The first row in square brackets gives, na³.

The second row is an Arithmetic Progression, with first term = 0 and common difference = 3a²𝛿x, giving,

The third row is,

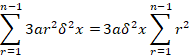
A common formula for the r² series is,

The fourth row is,

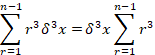
A common formula for the r³ series is,

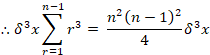
But

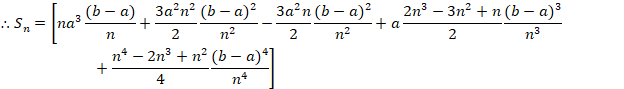
As n → ∞,

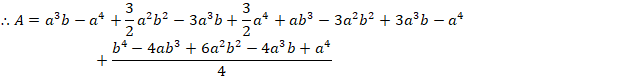
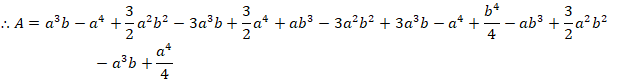
After subtractions and additions, we have,

If a is 0 and b is x,

Do we have to continue looking for the summation of each power series: x⁴, x⁵, x⁶, etc.? – No! Wait for the complete paper before the end of next month, which has my power series for xⁿ.

The Complete Paper

I will publish the complete paper, which solves different types of integrations, taught for pure and further math in Anglo-Saxon high schools, from First Principles; before the end of next month. I will publish the full paper in this website.

Chrys